We have spent some time learning how to graph circles, limacons, rose curves, and lemniscates on the polar coordinate plane. The material you see below is borrowed heavily from Yosh’s Graphing Polar Equations part 1, part 2, and part 3. I have included a link to the Word document instead of a pdf of the file in case you would like to modify the document to match her examples. A 3-page work sheet follows the notes sheet for practice and review of converting equations between polar and rectangular form.
Graphing Polar Equations Notes and Worksheet

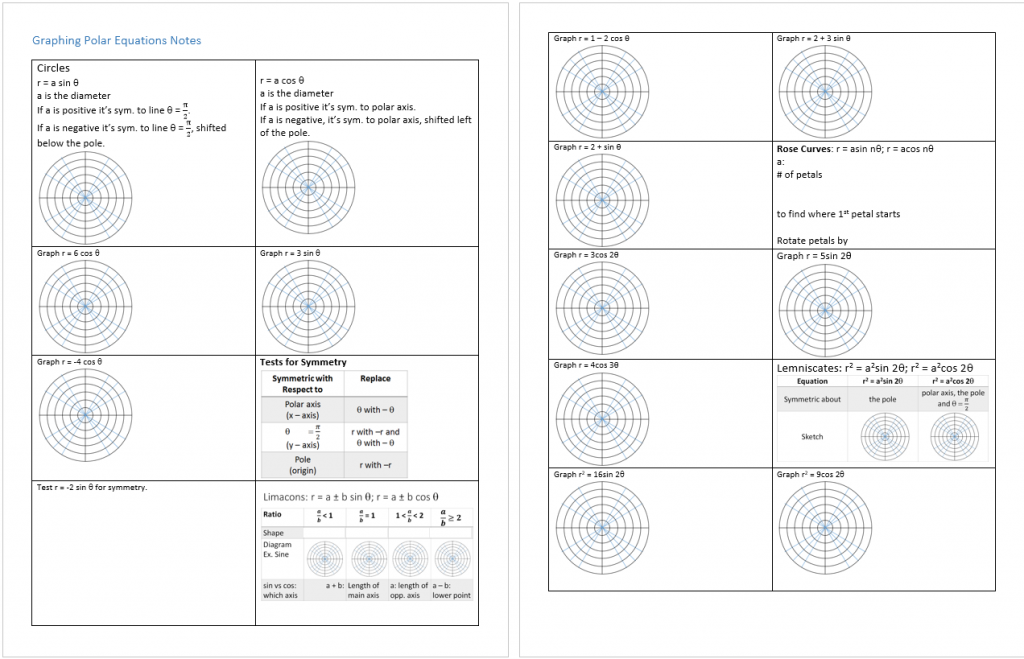

Do you have a way of explaining what these graphs are really used for in real life? Trying to teach it for the first time and lots of questions in how these graphs are used.
Polar equations are helpful when you need to work with things that are circular in nature. Converting between rectangular and polar is clunky when the equation is a line, but is beautiful when the equation is related to circles and curves. They are also used with things that involve rotations.